Talking Math in Ghirardelli Square

My stellar week of NCSM/NCTM fun ended with my family flying to San Francisco for a weeklong holiday. We started out at the Embarcadero, and ended up in Ghirardelli Square. We were sitting on a bench, eating our hot fudge sundaes, when my husband, Sam, started talking about the sale on big bags of Squares® inside.

He said he’d worked out the math, and big bags came out to about three Squares® per dollar. He was thinking about picking up a bag to take back to his office. I thought about grabbing one for Stenhouse, and one for my school. Conversation moved on. Eventually we all fell quiet.

Several minutes later, I was people-watching when my first grader, Daphne, asked, “Is it possible to break ten into three equal pieces?”

I asked why she was asking. (I do this often.)

“This is a question I have a lot. Like, today, when I wanted to buy my new doll, Olivia, it was some amount of money for three of them, and I wondered how much it would be for one of them, because I only wanted one.”

I was a little slow on the uptake. Long conference week. I sat there, blinking, for a minute. And then I said, “Oh! You’re asking about the chocolate, aren’t you?”

“Yeah. Because Daddy said they’re three for a dollar. What if you only wanted to buy one of them? How much would that be?”

“I see. But you didn’t ask if you could break a dollar into three equal parts. You asked if you could break ten into three equal parts. Where’d the ten come from?”

“Well, you know how there are ten 10s in 100?”

“I do.”

“It’s basically the same thing. Like, 6 + 4 = 10, and 60 + 40 = 100.”

I asked where the 100 came from.

“The 100 is because there are 100 pennies in a dollar. So if I figure it out for 10, then I’ll be able to figure it out for 100, because it’s basically the same thing.”

This is where I stopped her for a second so we could high-five. I mean, holy use of mathematical structure, Batman.

Daphne went on, “What I really need are ten things. Oh, rocks! Perfect!”

Daphne worked with the rocks for a long time. She was thinking hard. I kept her sister quiet, which is the challenge in moments like this.

Daphne finally said, “I don’t think I can make three equal pieces with rocks, because I can’t break this last rock apart. It works out to 3 + 3 + 4 or 3 + 3 + 3 + 1. I need a piece of paper to show you.” She grabbed the envelope I had in my bag.

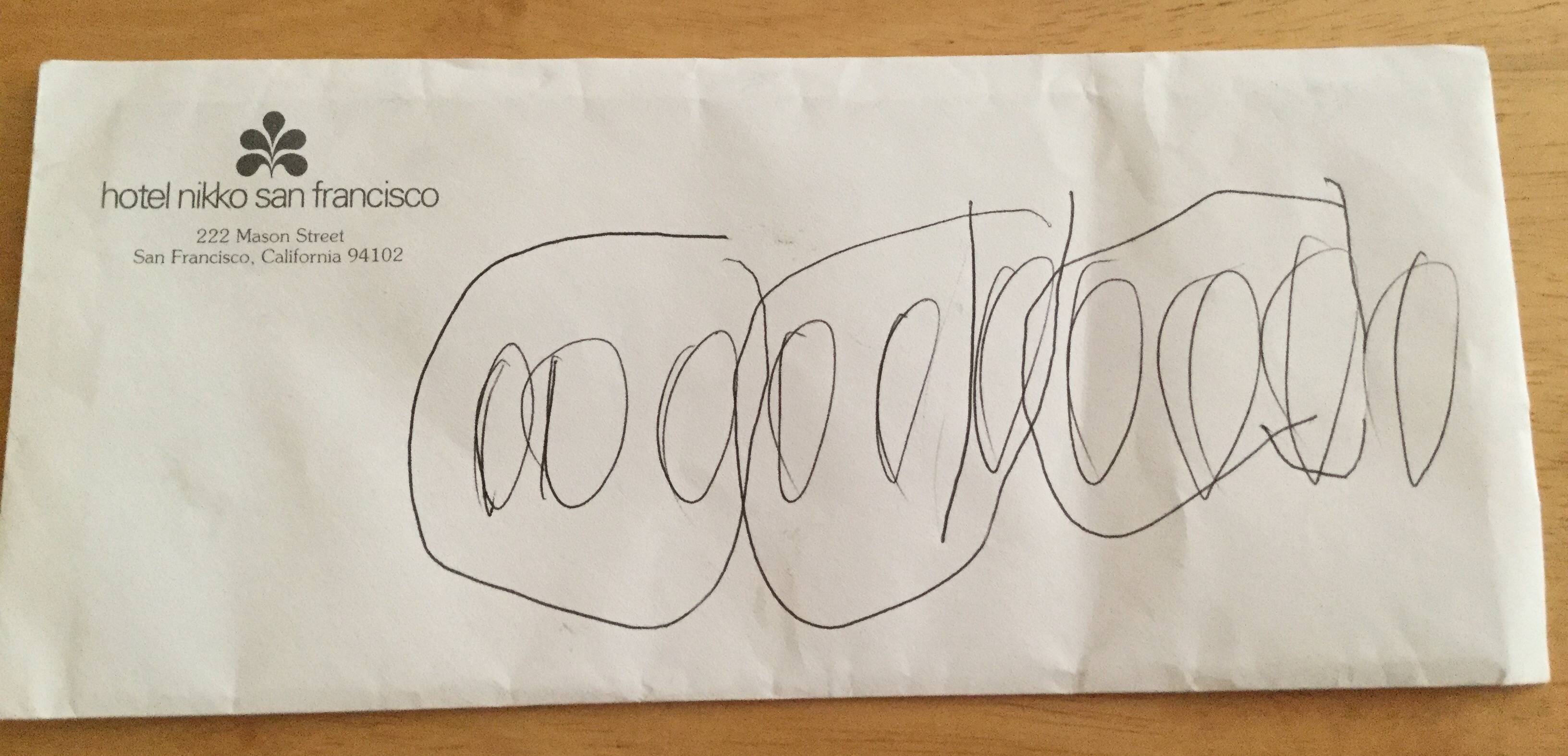
“See? It doesn’t work because I can’t break this rock! I can’t cut rocks into thirds.”

I asked, “What if they were crackers or cookies instead?”

“Then I could break up the last piece into three equal pieces. Then they’d each have three whole ones and one-third.”

“What do you mean, one-third?”

“Well, a third is one of three equal pieces.”

“What if you have three pieces, but they’re different sizes?”

“Then they’re not thirds. They’re just three chunks. I learned this from listening to you and Maya talk, by the way.”

Hmm. +1 for older sisters.

I asked, “So what do you think they do if they want to sell one piece of chocolate? How much should it be?”

She said, “With money, I should be able to to break it up. I can make change for the dollar. So I have 100 cents. So, 30 + 30 + 30 is 90, and that leaves 10 more…”

At this moment, she leapt up. “Wait! It’s the same thing again! It’s going to go on forever! With 100, it was 30 + 30 + 30 with 10 left. With 10, it’s 3 + 3 + 3 with 1 left. I can split up that 1 into three pieces, but there’s going to be a piece left. That one extra piece MAKES IT GO ON FOREVER! There’s always going to be an extra piece! Three, three, three!”

I happened to have a conference schwag calculator in my bag, and she got to see 100 ÷ 3.

“So how much should one piece of chocolate cost?”

“One-third of a dollar.”

“How much is that?”

“About 33 cents. If they charge 33¢, they get pretty close to a dollar.”

“How close?”

“Well, three chocolates would be 33 + 33 + 33, so 30 + 30 + 30 is 90, and 3 + 3 + 3 is 9, so that’s 99¢.”

I just spent a week thinking about the teaching and learning of mathematics with all kinds of amazing people. So much of the conversation is about how we can create the conditions so students do what Daphne did here:

• She noticed math in the world around her, and wondered about it.
• She posed an original (to her) mathematical question.
• She used structure to think about that question (in this case, the structure of place value).
• She used the strategy of solving a simpler problem.
• She looked for patterns and regularity.
• She stuck with her problem when it was hard for her.
• She used tools, representations, and models.
• She decontextualized and recontextualized the problem.
• She reasoned and justified.

I mean, she was all over the SMPs, right? And naturally, too. I wasn’t pushing any math at that moment, believe me. I was sitting there like a lump, very tired and very happy to be with my family. But I’ve done things at other times. Namely, I’ve made it clear to Daphne that math belongs to her. That her ideas are valuable. That I’m interested in them. That math involves asking questions. That she can figure things out for herself. That she owns the results of her investigations. That math is all around her. That she is a mathematician.

It’s paying off. Now, if only she can hold on to all of that.