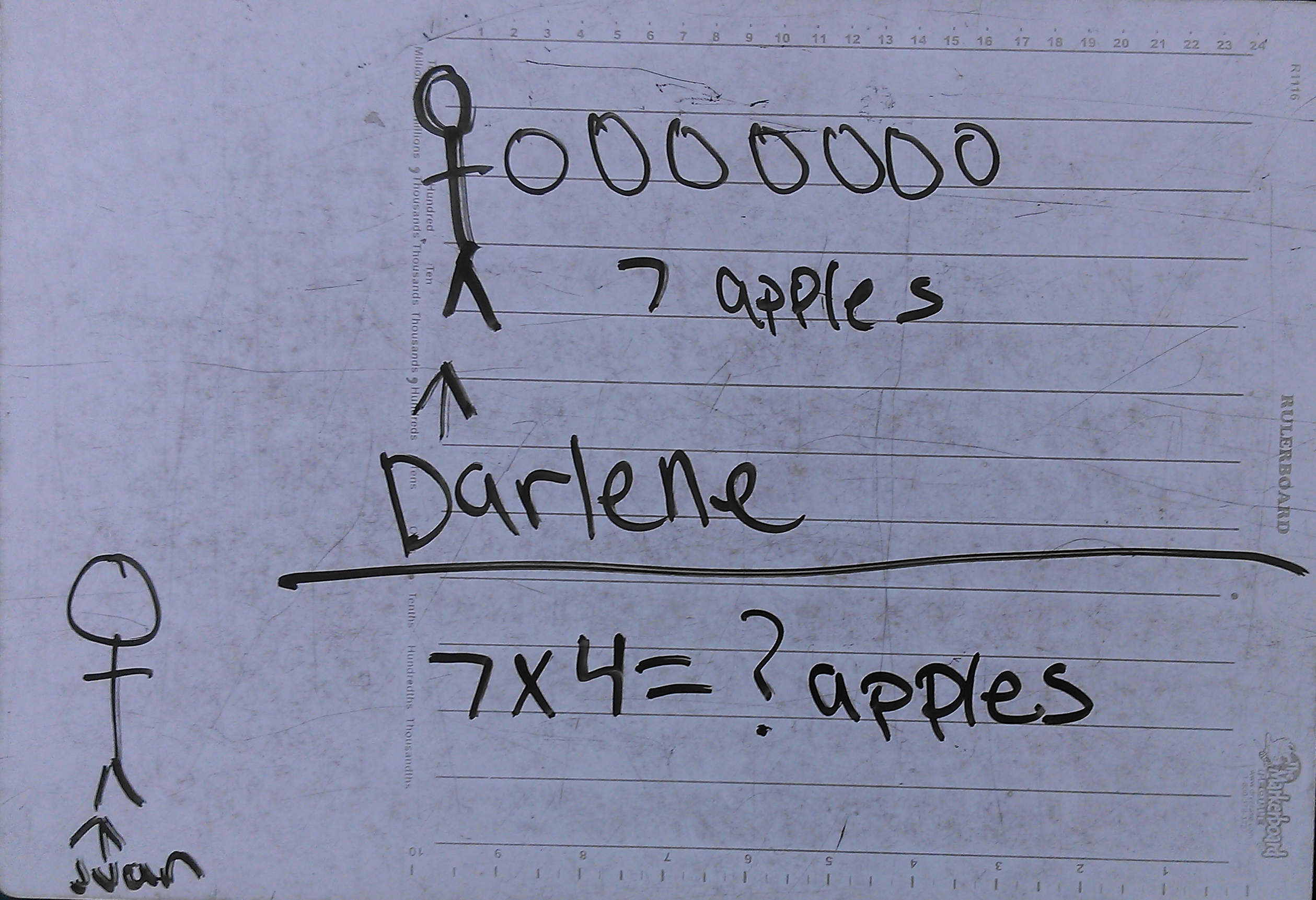Ask any elementary school teacher, and she or he will tell you that comparison problems are much harder for most kids than operations with other actions. For example, fourth-grade-teacher Jennifer Clerkin Muhammad asked her students to draw a picture of this problem from Investigations:
Darlene picked 7 apples. Juan picked 4 times as many apples. How many apples did Juan pick?
Her students are pros at representations and skillful multipliers, but we saw a lot of this:
Kids who were used to thinking of multiplication as either groups, arrays, or repeated addition had some productive, furrowed-brow time ahead of them. How can you represent comparisons? How is this multiplication? How do you draw the 4?
Then they started to get somewhere.
With each representation they discussed, Jen asked the excellent question:
“Where do we see the 4 times as many in this representation?”
Seriously. Jot that one down. It’s a keeper.
Jen and her class played with a series of problems involving multiplicative comparison, (height, quantity, distance, etc.). For each problem, she asked student to create a representation. And then she’d choose a few to discuss, asking, “Where is the ______ times as ______ in this representation?” Lightbulbs were going off everywhere as students broadened and deepened their understanding of multiplication.
Teachers often see the same struggle with comparison situations that can be thought of as subtraction or missing addend problems, like:
Marta has 4 stuffed animals. Kenny has 9 stuffed animals. How many more stuffed animals does Kenny have than Marta?
If kids have been taught “subtract means take away” or “minus means count backwards,” then this problem doesn’t fit the mold. And if kids have been taught keyword strategies, they’re likely to hear “more,” pluck those numbers, and add them together.
Knowing this as I do, I jump on opportunities to explore comparison problems with my own kids. I want them to have a fuller sense of the operations from the beginning, rather than developing too-narrow definitions that have to be broadened later. So, subtract doesn’t mean take away in our house. They’re not synonyms. I actually don’t worry about defining the operations at all: we just play around with lots of different types of problems, and I trust that my kids can make sense of them.
Yesterday, I was talking with Daphne about a neighbor visiting tomorrow. Our neighbor has a little girl, whom I’ll call Katrina. She’s about 2. We also have more awesome girls who live across the street. Let’s call them Lily, 15, and Gloria, 13. Gloria helps out once a week, and my girls idolize her. Here’s how the conversation kicked off:
Me: “If Katrina’s mother comes over to help me on Sunday, could you play with Katrina for a bit so her mom and I can work?”
Daphne: “Yes!!! We love playing with her. And she loves us. She cries when she has to leave us. We’re her best friends.”
Me: “I think Katrina feels the way about you and Maya that you and Maya feel about Lily and Gloria. It’s fun to hang out with older kids. You learn a little about what’s coming for you.”
Daphne: “Yeah. But for Katrina, Lily and Gloria would be TOO old. Like, she’d think they were grown-ups because they’re SO much older than she is. We’re older than Katrina, but we’re less older than Lily and Gloria are.”
So there’s my opportunity. She was comparing already. With a little nudge, I could encourage her to quantify and mathematize this situation. I thought I’d start with the simplest numbers so she could think about meaning first.
Me: “How much older are you than Katrina?”
Daphne: “I think she’s 2. So…”
There was a pretty good pause.
Daphne: “Are we counting on here?”
That’s paydirt.
Me: “What do you think?”
Daphne: “I think so. Because I want to know how many MORE. So, 2. 3, 4, 5, 6. I’m 4 years older than she is. Yeah. That makes sense.”
Me: “OK. So how many years older is Maya than Katrina?”
Daphne: “6.”
Me: “You didn’t count on that time. What did you do?”
Daphne: “Well, I knew that I was 4 years older than Katrina, and Maya is 2 years older than me, so 4, 5, 6. Because Maya is OLDER than me, I knew they had to be farther.”
Me: “What do you mean farther?”
Daphne: “Like, it had to be more. I was starting with 4, and then it had to be more farther apart.”
Me: “Oh! So you were trying to figure out whether to go 2 more or 2 fewer?”
Daphne: “Yeah. And it had to be 2 more, because Maya’s more older than Katrina than I am. I mean, I’m older than Katrina, and Maya’s older than me, so she’s more older than Katrina than I am. It had to be 2 MORE.”
Hot damn!
Daphne’s life might have been easier right here if she had some tools she doesn’t have yet. I’m thinking about number lines, symbols, and a shared mathematical vocabulary including words like distance or difference. Those tools might help her keep track and facilitate communicating her thinking to somebody else. But I love that we were able to have this conversation without those tools, while driving in the car where I couldn’t even see her gestures. Her thinking is there, and clear. The tools will come as a relief later on, when the problems are more complicated.
We kept going.
Me: “OK. So you’re 4 years older than Katrina and Maya’s 6 years older than Katrina. I wonder how that compares to Gloria? Is she about the same amount older than you two as you two are older than Katrina?”
Daphne: “Well, she’s 13. This is gonna be hard.”
Long pause.
Daphne: “She’s 7 years older than me.”
Me: “How’d you figure that out?”
Daphne: “I went 6. 7, 8, 9, 10, 11, 12, 13.” She held up her fingers to show me.
Me: “So how much older is Gloria than Maya?”
Daphne: “Umm…so Maya’s 8…umm…5. She’s 5 years older than Maya.”
Me: “How do you know?”
Daphne: “Because Maya’s 2 years older than me, so it’s 2 closer.”
This is the kind of problem where kids lose track of their thinking easily. Do we add or subtract that 2? In the last problem, she’d added. This time she subtracted. And she knew why!
When I glanced at Daphne in the rearview mirror, she had this awesome look on her face. She was looking out the window, but not seeing what was outside because she was so deep in thought. I wish I could have peeked inside her mind, and seen what she was seeing. Because, to use Jen’s question, where do we see the 2 years older? How did she see it? There was no time to ask, because she was onto pulling it all together.
Daphne: “So, Gloria’s a little bit more older than us than we are older than Katrina, because Gloria is 5 and 7 years older, and we’re 4 and 6 years older. But it’s pretty close.”
We pulled into the school parking lot on that one.
In our family, we count and manipulate objects all the time. Counting more abstract units like years, though, gives me a chance to open the kids’ thinking a little bit. In this conversation, Daphne was both counting and comparing years. Tough stuff, but oh so good.